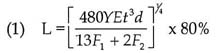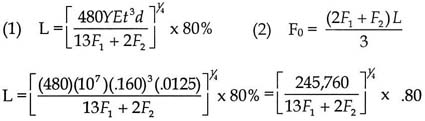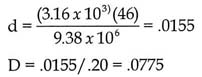How to Calculate Fastener Spacing

One of the great advantages of our spiral design is that the ratio of force to deflection is linear. That is, if it takes one pound to deflect the gasket 1% of its diameter, it will take 25 pounds to deflect the gasket 25% of its diameter. This allows fastener spacing to be easily calculated to fairly close tolerances.

If you’ve already chosen a gasket, these formulas can be used to determine fastener type and spacing in your application. However, if you’re not sure whether to employ a standard, moderate or low force gasket, these formulas can give you information that may help you determine which gasket will be the most cost effective.

Application Information

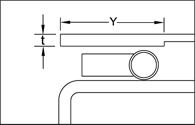
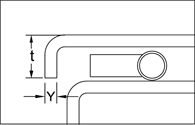
Formula 1 below can be used to calculate fastener spacing for all our gaskets, with the special considerations shown for certain types of gaskets. This formula determines fastener spacing along a straight edge. Corners require tighter spacing because of the increased stress placed on the cover at those points.

Fasteners

In this context, fasteners, include screws and any other means of attachment that will exert enough force to compress the gaskets 25% of their diameter. Care must be exercised to ensure this is true. The force each fastener must exert is equal to F0 as shown in Formula 2 (below).

Calculating Fastener Spacing

The Formulas

The formulas shown here are derived directly from classical beam problems.
Sample calculations are shown below in Example 1 and Example 2.

Note: We multiply by 80% as a safety factor.

Variables

All dimensions are in inches unless otherwise noted.

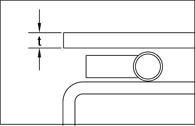
L = length between fasteners Y = width of cover edge (Assume 1″ if not distinct) E = modulus of elasticity of cover plate (psi) = 107 psi for aluminum = 3 x 107 psi for steel t = thickness of cover edge d = deflection of gasket = .022″ for Spira Cell = .20D” for all other gaskets D = diameter of spiral F1 = minimum force of gasket on cover (pounds/inch) F2 = maximum force of gasket on cover (pounds/inch) F0 = force fastener must exert to compress gasket 25% of its diameter

Both the “Y” and “t” are distinct in the top two illustrations Since “Y” is not distinct, we assume it to be 1″

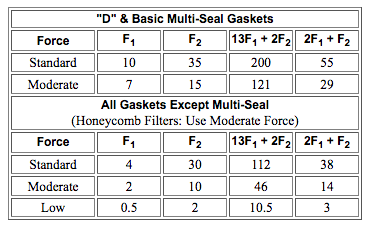
Force Data

Example #1

Application

Assume we need to shield an aluminum box that measures 10” by 12” and we have decided to use the groove mount Spira-Shield gasket in the -04 diameter. One of our design priorities is maintainability. If possible, we want to use quarter turn fasteners to quickly get in and out of the box.

Variables

Formulas

Calculations

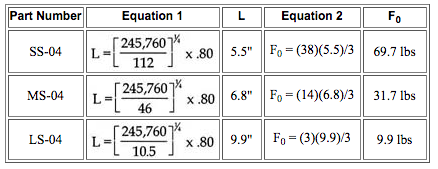
Fastener Spacing

At first glance, it seems that we might be able to use as few as six fasteners on the box if we use the LS-04 gasket. However, we must consider that the corners require tighter spacing than the straight portions of the box. So, on the straight sections, we can use the calculated spacing, while making sure that the corners are adequately secured. Recommendations for each gasket follow.

SS-04: Use three fasteners on each side for a total of 12 fasteners.
MS-04: Use three fasteners on the 12″ side and two fasteners on the 10″ side for a total of 10 fasteners.
LS-04: Use two fasteners on the 12″ side and two fasteners on the 10″ side for a total of 8 fasteners.

Design Analysis

SS-04: Acceptably wide spacing but requires a 70 pound fastener load which makes the use of quarter turn fasteners impossible.

MS-04: Acceptably wide spacing but requires a 32 pound fastener load and the maximum load our quarter turn fastener can supply is 25 pounds. However, we can take Equation 2 and work backwards using our knowledge of the maximum load of a quarter turn fastener.
So, we take the F₀ equation, set it equal to 25 pounds and solve for “L”.

25 = 14L/3
L=5.4″

With this additional constraint, the fastener spacing is reduced to 5.4″ which will require using three fasteners on each side for a total of 12 fasteners but will allow us to use quarter turn fasteners.

LS-04: Acceptably wide spacing and requires only a 10 pound fastener load which can be easily achieved using quarter turn fasteners.

Solution

Either the MS-04 with 12 fasteners or the LS-04 with 8 fasteners will work well with quarter turn fasteners. At this point, our decision must be based on cost versus convenience factors. The MS-04 gasket is more durable, slightly lower in price and will yield slightly higher shielding. The final determination is based on answering the question of whether the cost of four additional fasteners, in terms of time and dollars, is worth the benefits of using the MS-04 gasket.

Example #2

Application

Assume we need to shield an aluminum box, and we have decided to use a groove mounted “MS” series Spira-Shield gasket with 6.0 inch fastener spacing. What is the minimum size standard gasket we can use?

Variables

Formula

Calculation

Solution

The MS-05 Spira-Shield gasket has a diameter of .078″. Therefore, the minimum size Spira-Shield gasket which can be used is an MS-05.